Multiple Time Steps Algorithms for the Isothermal-Isobaric
Ensemble
The integrators developed in the previous section generates dynamics
in the microcanonical ensemble where total energy, number of particles
and volume are conserved. The derivation based on the Liouvillean and
the corresponding propagator, however lends itself to a
straightforward generalization to non microcanonical ensembles.
Simulations of this kind are based on the concept of extended system
and generate trajectories that sample the phase space according to a
target distribution function. The extended system method is reviewed
in many excellent textbooks and papers
[12,86,87,88,89,90,91,25]
to which we refer for a complete and detailed description. Here it
suffices to say that the technique relies on the clever definition of
a modified or extended Lagrangian which includes extra degrees
of freedom related to the intensive properties (e.g. pressure or
temperature) one wishes to sample with a well defined distribution function.
The dynamics of the extended system is generated in the microcanonical ensemble
with the true 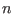 degrees of freedom and, additionally, the extra degrees of
freedom related to the macroscopic thermodynamic variables.
With an
appropriate choice, the equations of motion of the extended system
will produce trajectories in the extended phase space generating
the desired equilibrium distribution function upon integration
over the extra (extended) variables.
There are several extended system techniques
corresponding to various ensembles, e.g. constant pressure in the
NPH ensemble simulation with isotropic [92] and
anisotropic [93] stress, constant temperature
simulation [94] in the NVT ensemble and isothermal-isobaric
simulation [95] in the NPT ensemble.
As we shall see, the dynamic of the real system generated
by the extended system method is never Hamiltonian.
Hence, symplecticness is no longer an inherent property of the
equations of motion.
Nonetheless, the Liouvillean formalism developed in the
preceding section, turns out to be very useful for the derivation of
multiple time step reversible integrators for a general isothermal-isobaric
ensemble with anisotropic stress, or N PT3.1. This extended system is the most general among all
non microcanonical simulations: The NPT, NPH the NVT and even NVE
ensemble may be derived from this Lagrangian by imposing special
constraints and/or choosing appropriate parameters
[25,27]
degrees of freedom and, additionally, the extra degrees of
freedom related to the macroscopic thermodynamic variables.
With an
appropriate choice, the equations of motion of the extended system
will produce trajectories in the extended phase space generating
the desired equilibrium distribution function upon integration
over the extra (extended) variables.
There are several extended system techniques
corresponding to various ensembles, e.g. constant pressure in the
NPH ensemble simulation with isotropic [92] and
anisotropic [93] stress, constant temperature
simulation [94] in the NVT ensemble and isothermal-isobaric
simulation [95] in the NPT ensemble.
As we shall see, the dynamic of the real system generated
by the extended system method is never Hamiltonian.
Hence, symplecticness is no longer an inherent property of the
equations of motion.
Nonetheless, the Liouvillean formalism developed in the
preceding section, turns out to be very useful for the derivation of
multiple time step reversible integrators for a general isothermal-isobaric
ensemble with anisotropic stress, or N PT3.1. This extended system is the most general among all
non microcanonical simulations: The NPT, NPH the NVT and even NVE
ensemble may be derived from this Lagrangian by imposing special
constraints and/or choosing appropriate parameters
[25,27]
Subsections
procacci
2021-12-29